When it comes to math, there are a lot of concepts that can be confusing, but there's one particular formula that can be really helpful to know: the definite integral formula. This formula is used to calculate the exact amount of area under a graph, and it's an important concept to understand if you want to do well in calculus or other advanced math classes.
Definite Integral Formula
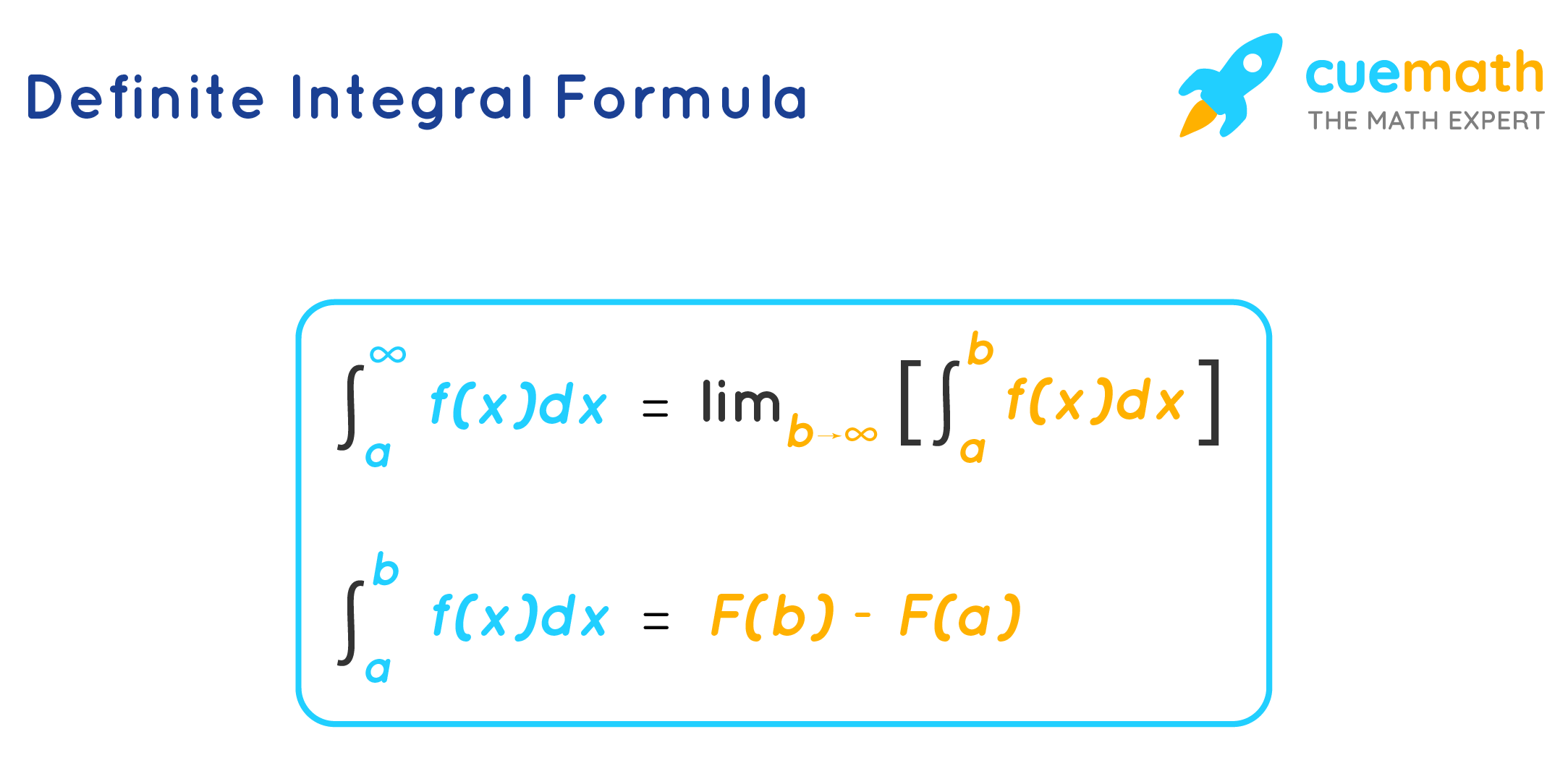
The definite integral formula looks like this: ∫[a,b] f(x)dx. The parts of the formula include:
- The symbol "∫", which stands for "integral".
- The numbers "a" and "b", which represent the limits of integration. These tell you what part of the graph you're looking at.
- The function "f(x)", which refers to the actual equation you're integrating.
- "dx", which is the differential. This tells you what variable will be changing as you integrate.
By plugging in the appropriate numbers and solving the equation, you can find out the exact area under the graph. It might seem complicated at first, but with a bit of practice, you'll soon be able to understand how to use the formula.
Example of Using the Definite Integral Formula
Let's say you have a graph like the one above. A definite integral formula that would be used to find the shaded area would look something like this: ∫[0,3] (x^2)dx.
You would integrate the function (x^2) with respect to x from 0 to 3. This would involve finding the antiderivative of (x^2), which is (x^3/3). Then, you would plug in 3 and 0 for x and subtract the two answers.
The final answer would be 9.
Practice Using the Definite Integral Formula
If you're looking to improve your understanding of the definite integral formula, there are a few things you can do. First, practice integrating different functions using the formula. You can also work through practice problems to see how the formula can be applied to real-world situations.
It's also a good idea to review the basic concepts of calculus, including limits, derivatives, and integrals. The more you understand about the subject, the easier it will be to work with the definite integral formula.
Overall, if you're interested in advanced math or science fields, knowing how to use the definite integral formula is incredibly important. Whether you're studying for a test or just want to get a better grasp on calculus, taking the time to practice and understand this formula can make a big difference in your success.
If you are searching about Definite Integral Formula - Learn Formula to Calculate Definite Integral you've visit to the right page. We have 6 Pictures about Definite Integral Formula - Learn Formula to Calculate Definite Integral like Integral Calculator With Steps / Triple Integral dz, dy, dx Solver, Definite Integral - YouTube and also Definite Integrals - YouTube. Here it is:
Definite Integral Formula - Learn Formula To Calculate Definite Integral
www.cuemath.com definite formula calculate dx
Definite Integrals - YouTube
www.youtube.com definite integrals
Calculus: The Definite Integral - YouTube
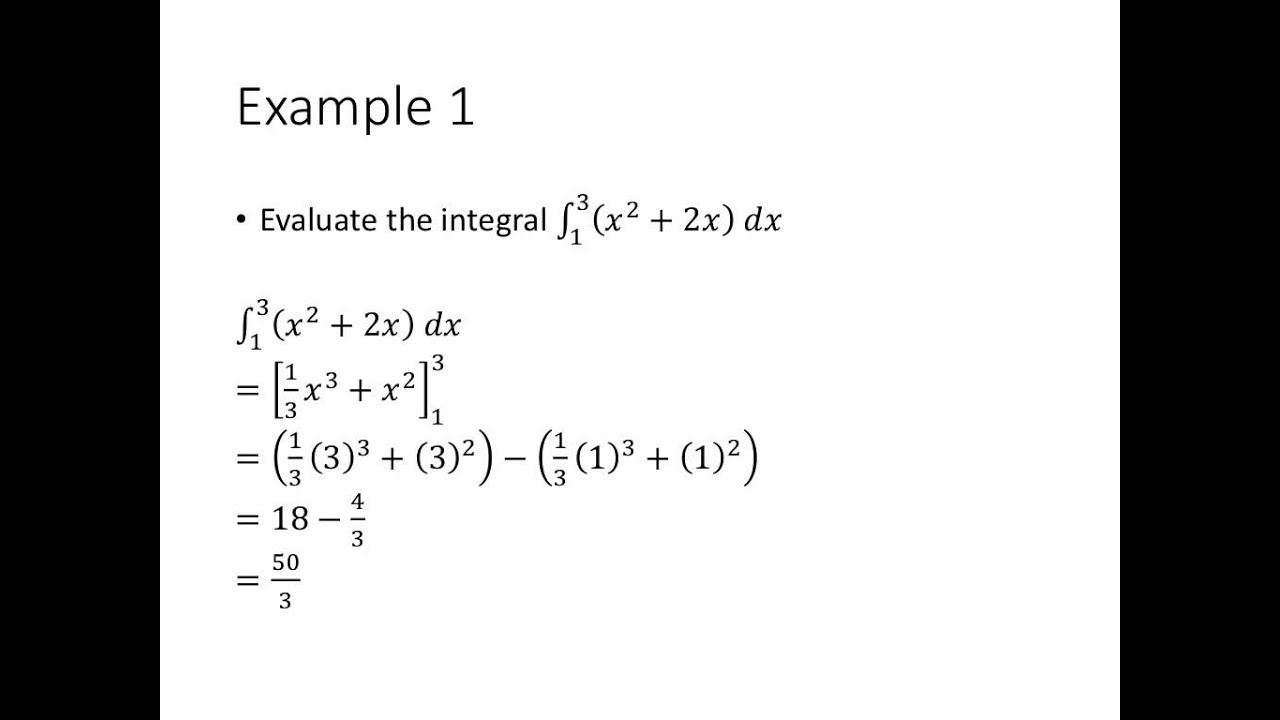www.youtube.com integral definite calculus
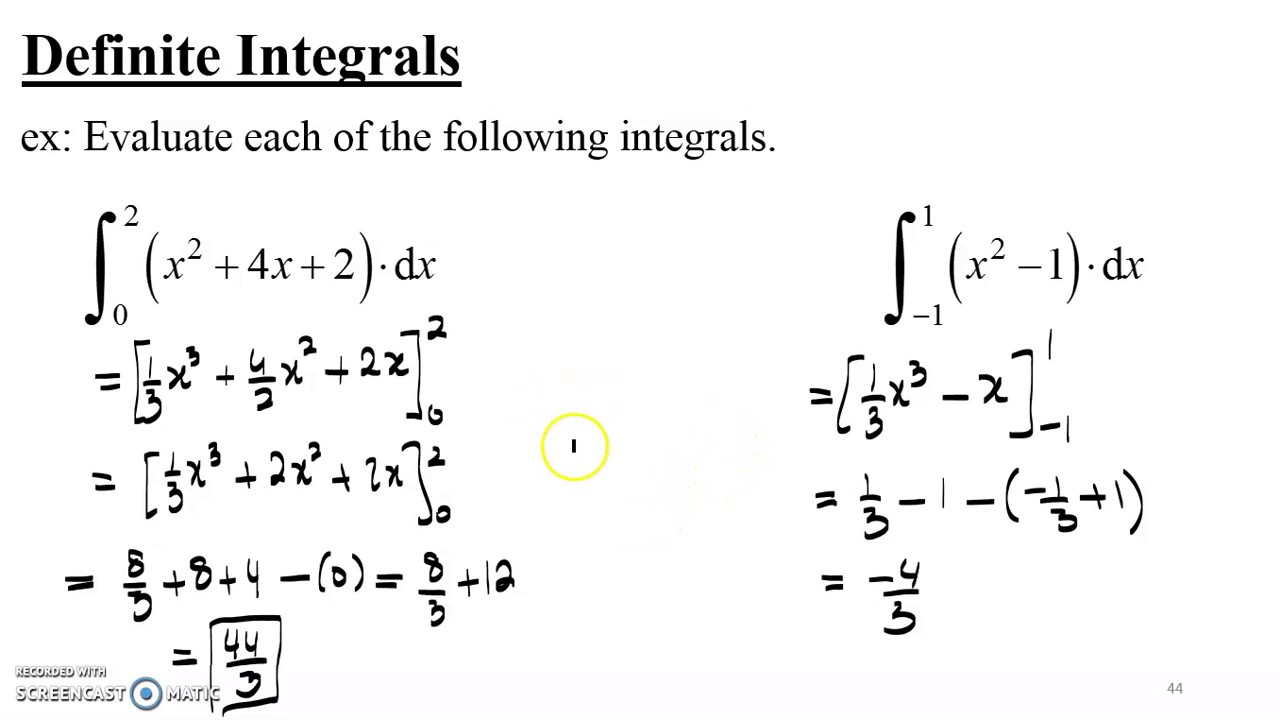
Calculating The Definite Integrals Associated With Two Regions
www.researchgate.net definite integrals calculating
Definite Integral - YouTube
www.youtube.com definite integral integration examples calculus basic
Integral Calculator With Steps / Triple Integral Dz, Dy, Dx Solver
uwekur.blogspot.com calculus definite integrals solver moreover entered capable sinx
Calculating the definite integrals associated with two regions. Definite integrals. Definite integrals calculating
Keine Kommentare:
Kommentar veröffentlichen